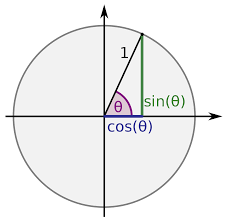
Introduction to Trigonometry

Proof:
↪ We consider a triangle ABC then we can have three possible figures where angle C is acute in figure i, right angle in figure ii and obtuse in figure iii.
From A draw AD perpendicular to BC ( Produce BC if necessary )
In ΔABC, AB2 = AD2 + BD2
⇒c2 = AD2 + BD2 → Ⅰ
In figure (i),
⇒⇒ AD = bSinC
Also,
⇒ DC = ACCosC = bCosC
⇒ BD = BC - DC = a-bCosC
Now from equation Ⅰ,
c2 = AD2 + BD2 (becomes)
c2 = (bSinC)2 + (a-bCosC)2
c2 = b2Sin2C + a2 - 2abCosC + b2CosC2
c2 = b2 + a2 - 2abCosC
⇒ 2abCosC = b2 + a2 - c2
⇒
Similarly in figure ii,
AB2 = BC2 + AC2
c2 = a2 + b2 = a2 + b2 - 2abCosC
2abCosC = a2 + b2 - c2
⇒
And in figure iii,
⇒
⇒ AD = bSinC
Also,
⇒
⇒ CD = -bCosC
⇒ BD = BC + CD = a - bCosC
Now from equation Ⅰ,
c2 = AD2 + BD2
= (bSinC)2 + (a-bCosC)2
= b2Sin2C + a2 + b2Cos2C - 2abCosC
= b2 + a2 - 2abCosC
⇒ 2abCosC = b2 + a2 - c2
⇒
Finally in all figures, it is seen
Similarly we can show