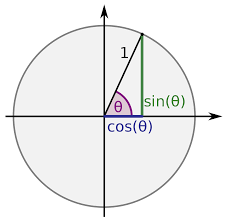
Introduction to Trigonometry

⁕ Statement: In any ΔABC, where R is the Circum-Radius of ΔABC.
Proof:
↪ Consider the circum-circle of ΔABC with center O and radius R. Then we can have three possible figures.
Where the angle A is acute in fig i, or right angled in fig ii or obtuse in fig iii.
Let O be the center of the circum-circle of ΔABC and R be the circum-radius. Join BO and produce it to meet the circle at D.
Now in figure i,
BAC = BDC = A, BCD = 90º (angle at the semicircle).= Sin(BDC)
↣= SinA
↣ 2R
In fig iii,
↣1 = Sin90º = SinA
↣ SinA ( Since BC = BD )
↣ SinA
↣ 2R
In fig iii, SinA
↣ SinA
↣2R
Therefore in each figure it is found that 2R
Similarly we can prove that:
Combining these we get, Proved.