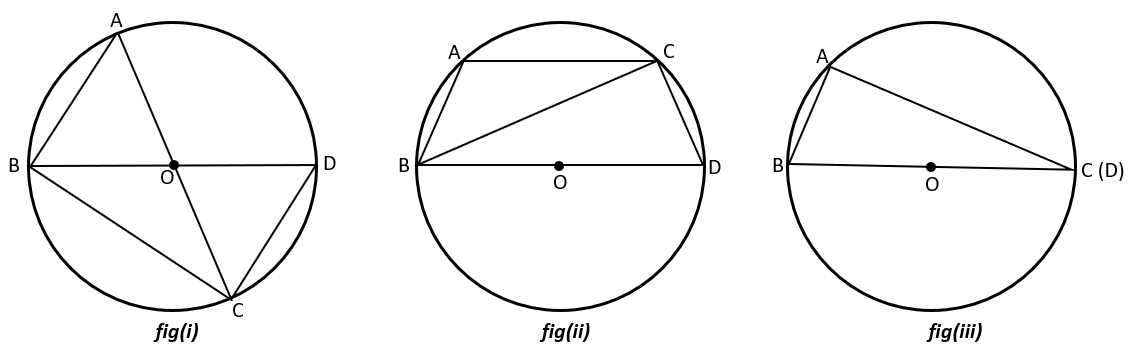
Let ABC be the triangle with all three vertex on the circumference of the circle with center O as shown in fig above.BO is drawn if the angle is either acute or obtuse as shown in fig(i) and fig(ii). Also, BO is produced to D to form the diameter BD as shown in fig. [C coincides D in fig (iii)]Then, circum-radius=OB=R(radius of circle) and BD=2R and angle BCD=90
0 [in fig (i) and (ii)] being angle at semi-circle. To Prove:
 1. For a triange as shown in fig(i)
1. For a triange as shown in fig(i)
BAC=BDC
[angle subtended by same segment]i.e., angle A = angle D.........(i)In triangle BDC,
 2. For a triangle as shown in fig(ii)
2. For a triangle as shown in fig(ii)

In triangle BDC,
 3. For a triangle as shown in fig(iii)
3. For a triangle as shown in fig(iii) BC = 2R = 2R sin A
[A=900 , sin A = sin 900 = 1]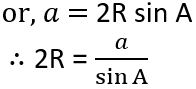
Hence, for every condition we have,
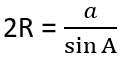
Similarly, we can prove,

Combining these we get,

, which is the Sine Law in terms of circum-radius.




 In triangle BDC,
In triangle BDC, 3. For a triangle as shown in fig(iii)
3. For a triangle as shown in fig(iii)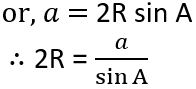
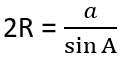 Similarly, we can prove,
Similarly, we can prove,  Combining these we get,
Combining these we get, , which is the Sine Law in terms of circum-radius.
, which is the Sine Law in terms of circum-radius.