Integration of rational fraction

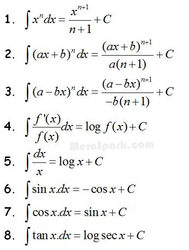
Integration of rational fraction:

In order to convert improper rational function into a proper one, we can use long division:

$$\frac{P\left(x\right)}{Q\left(x\right)}=F\left(x\right)+\frac{R\left(x\right)}{Q\left(x\right)},$$

where $F\left(x\right)$ is a polynomial, $\frac{R\left(x\right)}{Q\left(x\right)}$ is a proper rational function.

To integrate a proper rational function, we can apply the method of partial fractions.

This method allows to turn the integral of a complicated rational function into the sum of integrals of simpler functions.

The denominators of the partial fractions can contain non-repeated linear factors, repeated linear factors, non-repeated irreducible quadratic factors, and repeated irreducible quadratic factors.

To evaluate integrals of partial fractions with linear or quadratic denominators, we use the following $6$ formulas:

$$1.\int \frac{Adx}{ax+b}=A\ln \left|ax+b\right|$$

$$2.\int \frac{Adx}{{\left(ax+b\right)}^k}=\frac{A}{a\left(1-k\right){\left(ax+b\right)}^{k-1}}$$

For partial fractions with irreducible quadratic denominators, we first complete the square:

$$a{x}^2+bx+c=a\left[{\left(x+\frac{b}{2a}\right)}^2+\frac{4ac-b^2}{4a^2}\right].$$

Hence, we can write:

$$\int \frac{Ax+B}{{\left(a{x}^2+bx+c\right)}^k}dx=\int \frac{At+B^{\prime }}{{\left[a\left(t^2+m^2\right)\right]}^k}dt=\frac{1}{a^k}\int \frac{At+B^{\prime }}{{\left(t^2+m^2\right)}^k}dt,$$

where

$$t=x+\frac{b}{2a},m^2=\frac{4ac-b^2}{4a^2},B^{\prime }=B-\frac{Ab}{2a}.$$

The possible cases for fractions with quadratic denominators are covered by the following integrals:

$$3.\int \frac{tdt}{t^2+m^2}=\frac{1}{2}\ln \left(t^2+m^2\right)$$

$$4.\int \frac{dt}{t^2+m^2}=\frac{1}{m}\mathrm{tan-1}\frac{t}{m}$$

$$5.\int \frac{tdt}{{\left(t^2+m^2\right)}^k}=\frac{1}{2\left(1-k\right){\left(t^2+m^2\right)}^{k-1}}$$

Finally, the integral $\int \frac{dt}{{\left(t^2+m^2\right)}^k}$ can be evaluated in $k$ steps using the reduction formula

$$6.\int \frac{dt}{{\left(t^2+m^2\right)}^k}=\frac{t}{2m^2\left(k-1\right){\left(t^2+m^2\right)}^{k-1}}+\frac{2k-3}{2m^2\left(k-1\right)}\int \frac{dt}{{\left(t^2+m^2\right)}^{k-1}}$$