Theorem of Limit
Theorem:To prove: lim sin θ = 1 where θ is measured in radian θ→0 θLet O be the centre of the...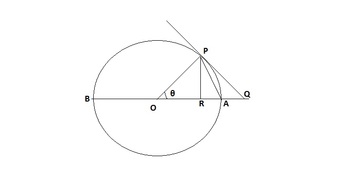
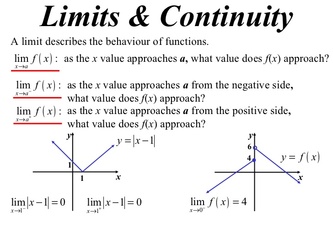
Limit and Continuity
Limit
A limit is defined as a number approached by the function as an independent function’s variable approaches a particular value.
For instance, for a function f(x) = 3x, you can say that “The limit of f(x) as x approaches 2 is 6”.
Symbolically, it is written as;
lim x->2 (3x) =3×2=6
Continuity
Many functions have the property that they can trace their graphs with a pencil without lifting the pencil from the paper’s surface. These types of functions are called continuous. Intuitively, a function is continuous at a particular point if there is no break in its graph at that point.First, a function f with variable x is continuous at the point “a” on the real line, if the limit of f(x), when x approaches the point “a”, is equal to the value of f(x) at “a”, i.e., f(a). Second, the function (as a whole) is continuous, if it is continuous at every point in its domain.
Mathematically, continuity can be defined as given below:
A function is said to be continuous at a particular point if the following three conditions are satisfied.
1. f(a) is defined
2. lim x->a f(x) exists
3.lim x->a+ f(x)=lim x->a- f(x)=f(a)
As mentioned before, a function is said to be continuous if you can trace its graph without lifting the pen from the paper. But a function is said to be discontinuous when it has any gap in between.
lim x->a+ f(x) is called right hand limit and lim x->a- f(x)is called left hand limit of f(x) at x=a.
