Theorem of Limit

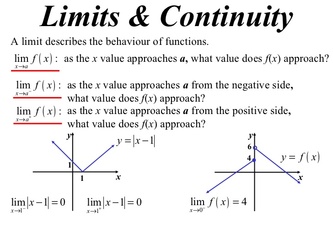
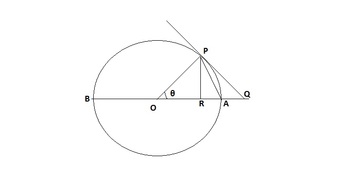
Theorem:To prove:
  lim     sin θ = 1        where θ is measured in radian                                                                                       θ→0      θ
Let  O be the centre of the circle and r be the radius. AP is an arc that subtends an angle θ at centre O. Let PQ be the tangent of the circle at P. BA is produced and joined at Q.
Join PA and draw PR perpendicular to BA. Now,
Area of △AOP ≤ Area of sector AOP ≤ Area of △POQNow, Area of △AOP = ½ OA. PR       [ OA = r ]
                           = ½ r. PR                                     = ½ r²sinθ        [ PR= rsinθ ]
Area of sector AOP = ½ r²θ
Area of △POQ = ½ OP. PQ       [ OP = r ]
                          = ½ r. PQ                          = ½ r²tanθ         [ PQ = r tanθ ]

∴   Area of △AOP  ≤  Area of sector AOP  ≤  Area of △POQ           ½ r²sinθ  ≤  ½ r² θ  ≤   ½ r² tanθ       Dividing by sinθ on all sides,                  1      ≤       θ     ≤        1                                                                                                                                                             sinθ           cosθ                  1       ≥        sinθ        ≥        cosθ                                                                                                                                                θApplying     lim   ,                                                                                                                                                                       θ→0
 lim   1   ≥   lim    sinθ      ≥       lim    cosθ                                                                                                   θ→0          θ→0    θ                 θ→0
  1         ≥                  lim    sinθ         ≥    1
                                θ→0     θ
∴  lim     sinθ         =   1
   θ→0      θ