Conic Section: Parabola

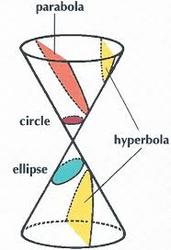
In this note, we will discuss:

• Parabola, 


• Some terminology, 


• Standard Equation of parabola and its different forms, 


• Equation of parabola with its axis parallel to x-axis, 


• General Equation of parabola, 


• A point and a parabola, 


• Condition that a line is tangent to a parabola, 


• Equation of tangent and normal to the parabola y² = 4ax at a point P(x₁,y₁)

Parabola:

A conic section is called a parabola if its eccentricity is unity, i.e. e = 1. 

OR,

A parabola is a locus of a point which moves in a plane such that the ratio of the distances from a fixed point and a fixed line in always unity.

i.e. it is at equidistance from a fixed point and from a fixed straight line.

Terminology

Focus : the fixed point

Axis of parabola : the perpendicular line which passes through the focus and vertex of the parabola

Focal distance : the distance between any point on parabola and the focus

Focal chord : any chord of the parabola passing through focus

Latus rectum : the focal chord perpendicular to the axis of parabola

[Image missing]

Standard Equation of parabola:

y² = 4ax

Let S(a,0) is focus of the parabola whose axis is x-axis and vertex at V(0,0). The equation of directrix is x + a = 0

Let P(x,y) be any point on the parabola. Join PS and draw PM perpendicular on the directrix of the parabola.

For parabola, 

            PS = PM

or,        PS² = PM²

or,       

or,        x² + y² - 2ax + a² = x² + 2ax + a²

or,        y² = 4ax           is the required equation.

    -        Latus rectum:

    Let L = (a,y’)

    ∴ y’² = 4a.a  ⇒ y’ = ± 2a

    ∴ L(a,2a) and L’(a,-2a) are the points of the latus rectum on parabola and length of latus rectum is 4a.

Different forms of standard equation

i.    y² = - 4ax

ii.    x² = 4ay

iii.    x² = - 4ay

Equation of parabola with its axis parallel to x-axis:

(y - k)² = 4a(x - h)

Let V(h,k) is vertex of parabola of which axis is parallel to x-axis.

Let S(h + a, k) is focus of parabola then, the equation of directrix is

x - (h - a) = 0

Let P(x,y) is any point on the parabola. Join PS and draw PM perpendicular on the directrix.

For parabola,

            PS = PM

or,       PS² = PM²

or,       

or,        x² - 2x(h + a) + (h + a)² + (y - k)² = x² - 2x(h - a) + (h – a)²

or,        (y - k)² = 2x[h + a - h + a] + (h - a)² - (h + a)²

or,        (y - k)² = 4ax – 4ah

or,        (y - k)² = 4a(x - h)

Similarly, the equation of parabola of which axis is parallel to y-axis is

(x - h)² = 4a(y - k)

General equation of parabola

(a’² + b’²){(x - h)² + (y - k)²} = (a’x + b’y + c’)²

Let S(h,k) be the focus of parabola of which equation of directrix is

a’x + b’y + c’ = 0

Let P(x,y) be any point on the parabola. Join PS and draw PM perpendicular on directrix

For parabola,

            PS = PM

or,        PS² = PM²

or,       

or,       (a’² + b’²){(x - h)² + (y - k)²} = (a’x + b’y + c’)²  is the required equation.

A point and a parabola:

Let P(x₁,y₁) be any point. Draw PM perpendicular to the axis of parabola.

∴ OM = x₁, PM = y₁ ___ⓐ

Let PM meet the parabola at point Q. Then,

QM² = 4ax₁___ⓑ

The point P lies outside or on or inside the parabola according as

            PM² > QM²

or,       PM² = QM²

or,        PM² < QM²

i.e.

            y₁² > 4ax₁

or,        y₁² = 4ax₁

or,       y₁² < 4ax₁

Condition that a line is tangent to a parabola:

Let y = mx + c ___ⓐ

            be a line and

y² = 4ax ___ⓑ

            be a parabola.
Solving ⓐ and ⓑ, we have

            (mx + c)² = 4ax

or,        m²x² + 2x(mc - 2a) + c² = 0 ___ⓒ

            which is quadratic in x-axis.

This gives two values of x and corresponding two values of y.

The line will be a tangent to the parabola if discriminant of equation ⓒ is zero i.e.

            4(mc - 2a) ² - 4m²c² = 0

or,        4a² - 4mca = 0

or,        4a(a - mc) = 0

∵ 4a ≠ 0

∴ a – mc = 0

or,        c = a/m            is the required condition.

∴ The line

            y = mx + a/m   is a tangent to the parabola y² = 4ax.

Equation of tangent and normal to the parabola y² = 4ax at a point P(x₁,y₁)

Let P(x₁,y₁) be a point on the parabola y² = 4ax and Q(x₂,y₂) be its neighboring point on the parabola.

            ∴ y₁² = 4ax₁

            and, y₂² = 4ax₂

Subtracting them,

            y₂² - y₁² = 4ax₂ - 4ax₁ 

or,      = Slope of PQ

Now,

Equation of chord PQ is

        

or,    

The chord PQ becomes tangent to the parabola at point P if

            Q → P i.e. y₂ → y₁ and x₂ → x₁

or,     

or,        yy₁ - y₁² = 2ax - 2ax₁

or,        yy₁ - 4ax₁ = 2ax - 2ax₁

or,        yy₁ = 2ax + 2ax₁

or,        yy₁ = 2a(x + x₁)

 

Now,

Slope of tangent = 2a/y₁

Slope of normal = - y₁/2a

Equation of normal to the parabola at a point P(x₁,y₁) is

            y - y₁ = - y₁/2a (x - x₁) ___ⓐ

Let m = - y₁/2a            ⇒ y₁ = - 2am

From y₁² = 4ax₁

or,        (- 2am)² = 4ax₁

or,          x₁ = am²

 

∴ From ⓐ

               y + 2am = m(x - am)²

or,       y = mx - 2am - am³      is the required normal in m form.

<Here is a link to my resource of Parabola made in Geogebra, which you might find it helpful.>

Geogebra - Conic Section Parabola

<If you find any difficulty or mistake, do reach out to me through the comment section or social media.>

<notes source: from Rakesh Kumar Jha (RK) sir and book>
<images made from Geogebra>