Quantum Numbers

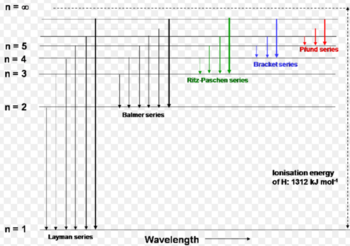
Quantum Numbers

    Quantum numbers are the set of four numbers which give complete information of particular electron relating to its size of energy level, shape and orientation of orbital with its spin.     Each electron in an atom is described by four different quantum numbers. They are:

1. Principal Quantum Number (n)

2. Azimuthal Quantum Number (l)

3. Magnetic Quantum Number (m)

4. Spin Quantum Number (s)

1. Principal Quantum Number (n):

    Principal quantum number was introduced by Neils Bohr (1913).

    Its value ranges from 1 to ∞.

    It gives information about the principal energy level of an electron. For the first energy level, closest to the nucleus n=1, for second energy level n=2 and so on. The various principal energy levels are also designated by the letters K, L, M ... and so on starting from the nucleus i.e 

    K < L < M < N ....

    1 < 2 < 3 < 4 ...

Information from Principal Quantum Number:

• It gives radius of n^th orbital. 


• It gives information about energy of n^th shell.


• It gives information about the maximum number of electrons that can be accumulate by particular shell (from 2n² rule).

2. Azimuthal Quantum Number / Subsidiary Quantum Number (ℓ):

    Azimuthal quantum number was introduced by Arnold Sommerfeld.

    For given value of n, the value of ℓ varies from 0 to (n-1). 

    It gives information about sub-shell or sub-energy level. Within the principal shell, it is found to be composed of closely packed discrete lines (hyper-fine lines) which is originated due to presence of different sub-shells with slight energy difference. The shape difference of different sub-shells of a shell gives rise to slight energy difference between them and to describe it, azimuthal quantum number was introduced. Sub-shells are designed as s, p, d and f.

Information from Azimuthal Quantum Number:

• It gives information about sub-energy levels.


• It describes the shape of different sub-shells.


• It describes about relative energy of sub-shell:  s < p < d < f


• It tells about maximum number of electron present in sub-shell (by 2[2l+1] ).

Values of ℓ	Sub-shells	Historical name	Maximum electron	Shape
0	s	Sharp	2	Spherical
1	p	Principal	6	Dumbbell
2	d	Diffuse	10	Double dumbbell
3	f	Fundamental	14	Complex / Unique

3. Magnetic Quantum Number (m):    Magnetic quantum number was introduced by Linde.    Its value lies from -ℓ to +ℓ. For provided value of ℓ, it has value (2ℓ+1).
    When the hyper-fine line spectrum is placed in strong magnetic field, it undergoes splitting to give number of fine lines with slight energy difference. In order to describe the origin of fine line spectrum in strong magnetic field, magnetic quantum number was introduced.      It distinguishes and explains about the orbitals of sub-shells.

Information from Magnetic Quantum Number:

• It gives information about number of orbitals in a sub-shell (by 2ℓ+1).
  


• It gives information about the orientation of orbitals in space. 

   
4. Spin Quantum Number (s):

    Spin quantum number was introduced by George Uhlenbeck and Samuel Goudsmit. 

    It can only have two values i.e +½ and -½, since the electron can spin either clockwise or anti-clockwise about its axis. 

    A clockwise electron spin gives upfield spin momentum denoted as ↑ and is described by s=+½. An anti-clockwise electron spin gives downfield spin momentum denoted as ↓ and is described by s=-½.

    Two electrons present in an orbital are denoted as ↑↓. 

    This quantum number helps to explain the magnetic properties of the substance (hence also symbolized as m_s). A spinning electron behaves like a micro magnet with a definite magnetic moment. If an orbital contains 2 electrons, the two magnetic moment opposes and cancel each other.