Mathematics Exam Paper ~ 2078

Rewrite the correct option in your answer sheet.

1. If f(x) = x² + kx + 1 for all x and f is an even function then the value of K is equal to
(a) 1 (b) 2 (c) 0 (d) -1

2. If A = [-2, 4) and B = (2, 5] then A ∩ B is
(a) [-2, 5]  (b) (2, 4)  (c) [-2, 2]  (d) (-2, 5)

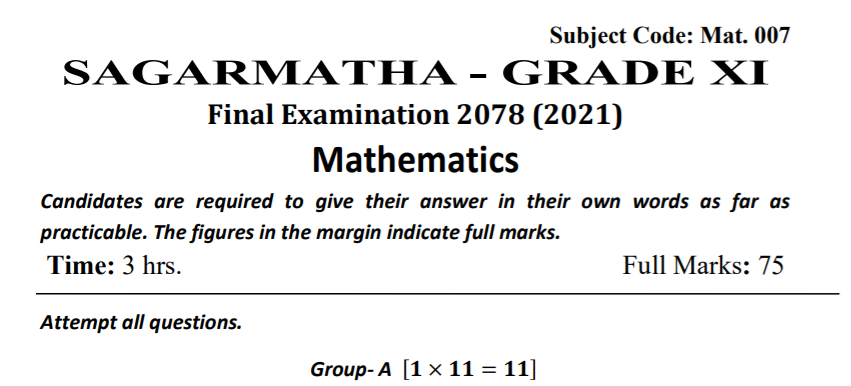
3. If a = √6, b=2, c = √3 - 1 then B is
(a) 45  (b) 30  (c) 60  (d) 135

4. The equation of diameter of the circle x² + y² - 6x + 2y = 0, which passes through the origin is 
(a) x + 3y = 0 (b) x - 3y = 0 (c) 3x + y = 0 (d) 3x - y = 0

5. The locus is known as parabola if the eccentricity (e) is
(a) equal to 1 (b) less than 1 (c) more than 1 (d) equal to zero

6.  then the projection of b on a is
(a) 3   (b) 4    (c) 5    (d) 6

7. Form the group of 10 items ∑x = 452, ∑x² = 24720 and mode = 43.7. Then the coefficient of skewness if
(a) -0.077  (b) 0.077   (c) 0.079    (d) - 0.079

8. The differentiating coefficient of cos-1x + sin-1x is equal to
(a) π/2  (b) 2/√(1-x2)    (c) 0  (d) none of these

9. The value of 
(a) 3x - 7 ln |x+2| + c    (b) 3x + 7 ln|x+2| + c   (c) 3x - 7 ln|3x-1| + c  (d) 3x + 7 ln|3x-1| + c

10. A body is dropped from tower of height 78.4 m. Then the time to reach the ground is 
(a) 6s  (b) 4s   (c) 3s   (d) 2s

OR

The demand function P = 12 - 0.5Q then the marginal revenue is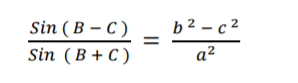
(a) 12-Q  (b) 12-PQ  (c) 12+Q  (d) 12 + PQ
11. . How many iterations do you need to get the approximate root of an equation f(x) = 0 by by bisection method if you start with initial guesses a = 1 , b = 2 and error tolerance 10^-4?(a) 12   (b) 10   (c) 2  (d) 14
"Group B" [ 5 × 8 = 40 ]
12. (a) If p and q are two statements then prove that:  p ⇒ q ≡ (~p v q) [3](b). Is the function f: N→N defined by f(x) = 3x bijective? [2]
13. (a) Find the square root of the complex number: -5 + 12i. [2](b) If a^x = b^y = c^z and a, b, c are in G.P. then show that x, y and z are in H.P. [3]
14. (a) In any triangle ABC, prove that [2]
(b) If two pair of straight lines represented x² - 2axy - 3y² = 0 and x² - 2bxy - y² = 0 are such that each pair bisects the angle between the other pair, prove that ab + 2 = 0. [3]
15. (a) Calculate the Karl Pearson's coefficient of skewness from the following data: [3]

Profit	below 80	below 90	below 100	below 110	below 120
No. of cost	12	30	65	107	130

b. A class consists of 30 boys and 20 girls. If two students are chosen at random, then find the probability that [2]
i. one is boy another is girl
ii. both are girls
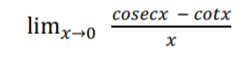16. a. Evaluate:  [2]b. Write the conditions that the graph of the function y = f(x) defined in an interval ( a, b ) is concave upward and concave downward. Find the maximum and minimum values of curve: y = 4x³ - 6x² - 9x + 1 on the interval (-1,2). Also, find the points of inflection.  [3]
17. a. Integrate:  [2]b. What does an indefinite integral mean? How does it differ from definite integral? Obtain the area bounded by the curves y = 4x and x = 4y.  [3]
18. Use simplex method to find the optimal solutions of the LPP:
Maximize: F = 2x + 5y subject to the constraints.
where x,y ≥ 0.  [5]
19. a. Resolve a force 30N into two components making angles 30° and 45° with its direction. [2]b. A stone is dropped into a well reaches the water with velocity 49m/s and sound of striking water is heard in 5⅓ seconds after it is let fall. Find the velocity of the sound. [3]OR19. a. If the marginal cost of product is given by C' (x) = 36 - 20x + 6x2, where x is the number  of units and initial cost is Rs 20, then find the total cost function and average cost function. [2]b. The demand function for a good is given as Q = 65 - 5P. Fixed the costs are Rs 30 and each unit produced costs an additional Rs 2. [3]i. Write the equations for total revenue and total costs interms of Q.ii. find the break-even points algebraically.iii. Graph the total revenue and total costs and hence estimate the break-even point.

"Group C" [ 8 × 3 = 24 ]

20. a. Find the domain and the range of the function: [ 4 + 4 = 8 ]

b. Draw the graph of the function including different characteristics: y = 2Sinx ( 0 ≤ x ≤ 2π )

21. a. Given a = √3 + 1, b = √6, c = √3 -1, solve the triangle ABC.     [ 3 + 3 + 2 = 8 ]
b. Show that the angle between the tangents to the parabola y² = 4x and x² = 4y at their points of intersection other than origin is tan^-1¾.
c. Prove vectorially that: a² = b² + c² - 2bcCosA

22. a. Find from the first principles the derivative of: [ 4 + 2 + 2 = 8 ]
b. A spherical balloon is inflated at the rate of 20cm³/sec. Find the rate of increasing its surface area when the radius is 8cm.
c. Evaluate: 

⁕⁕⁕