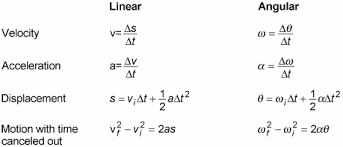
Important relations in Circular Motion

Centripetal Acceleration
↪ The acceleration due to a centripetal force is called centripetal acceleration.
↪ It is also known as a radial acceleration.
↪ In circular motion, when an object is moving at constant angular velocity, its linear velocity is continuously changing in direction but its magnitude remains constant.
↪ Therefore there must be a force acting toward the center of the circle called centripetal force which plays a role just to change the direction of the linear velocity of the object in circular motion.

Centripetal Force
↪ Centripetal force is a force acting on an object in circular motion so that it remains accelerating toward the center of the circle.
Expressions for Centripetal Acceleration and Centripetal Force
↪ Suppose an object of mass m is moving in a circle of radius r and center O at a constant linear velocity v and angular velocity ω.
↪ Suppose the object moves from point A to point B so that it describes an angular displacement θ about O.
↪ Since the object moves at constant speed, its speed at point A is v directed along the tangent AA" and its speed at point B is v directed along the tangent BB".
 It is clearly seen from the figure that ∠AOB = ∠BA'A" = ∠B"BB' = θ
It is clearly seen from the figure that ∠AOB = ∠BA'A" = ∠B"BB' = θ
∴ the change in velocity parallel to
AO = vsinθ – 0
and the change in velocity perpendicular to
AO = vcosθ – v
When θ becomes very small (i.e., B is very close to A),
then sinθ → θ and cosθ → 1.
∴ the change in velocity along AO, ∆v = vθ
and the change in velocity perpendicular to AO = v(1) – v = 0.
If the object in the circle takes a small time ∆t = t to bring the change in the velocity ∆v along AO, then the acceleration along AO,
↪ This is the centripetal acceleration because it is directed toward the center of the circle.
From F = ma, we have,

↪ This is the centripetal force.
Centrifugal Force
↪ The outward directional reaction force experienced by an object in circular motion is called the centrifugal force.
↪ It is a reaction force of the centripetal force.
↪ The magnitude of the centrifugal force acting on an object of mass m moving with uniform linear velocity v and angular velocity ω along a circular path of radius r is,
